Final Assignment
EMAT 6680 Summer 2012
Nicolina Scarpelli
Investigation 1:
(a) Prove Ceva's Theorem using ratio of Areas
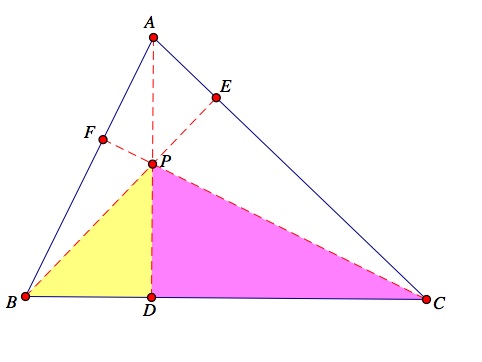
Definition: A cevian of a triangle ABC is a line segment joining a vertex of the triangle to a point on the opposite side. Thus, if D, E, and F are points on sides BC, AC, and AB of triangle ABC, then the line segments AD, CF, and BE are cevians.
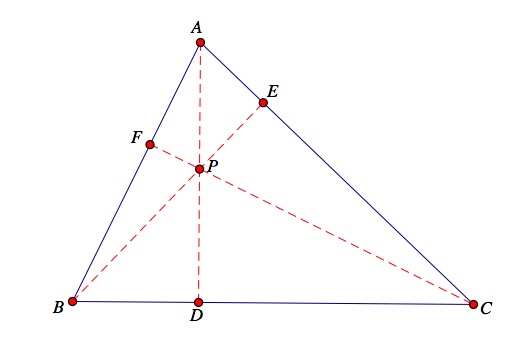
If the three Cevians AD, BE, and CF are concurrent at P, then prove:
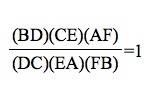
Let's assume AD, CF, and BE of triangle ABC are concurrent at a point P. Then, triangle BDP and triangle DPC have a common altitude to P from BC; thus, when two triangles share the same altitude the ratio of their areas are in proportion to their respective bases: BD and DC. Meaning, the ratio of their areas is equal to the ratio of their bases.
Similarly, triangle ABD and ADC have a common altitude to A from BC.
Thus, we can set up the proportion below:
QED
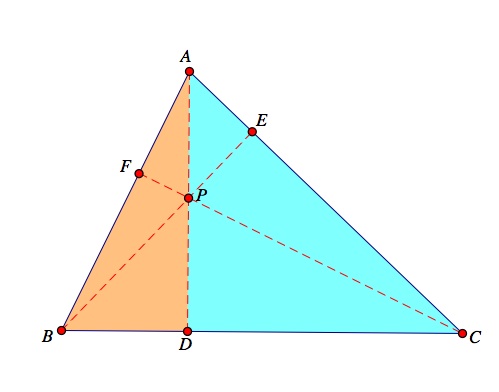
(b) Prove the Converse of Ceva's Theorem:
If
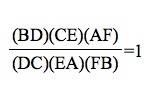
then the Cevians are concurrent.
Now, suppose
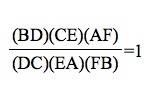
Then, let P be the point of intersection of AD and BE. Let CP meet side AB at a point F'.
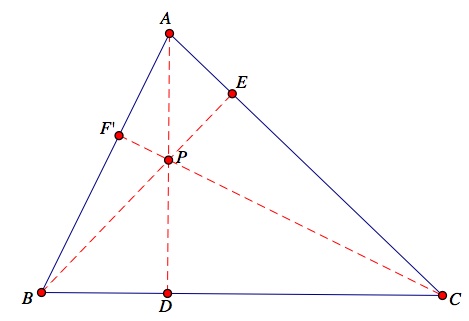
Then, by the forward argument, we have
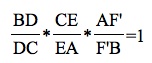
Therefore, we have two equations equal to 1 and we can set these equations equal to each other and solve.
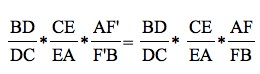
Thus,
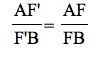
so that both F and F' divide AB in the same ratio and must therefore be the same point. Thus, the Cevians are concurrent.
QED
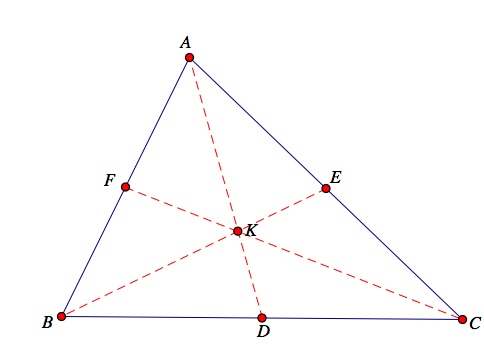
(c) Use the converse of Ceva's Theorem to prove the medians of a triangle are concurrent.
Consider the triangle ABC below with E being the midpoint of side AC, F being the midpoint of side AB, and D being the midpoint of side BC.
Medians connect vertices with the midpoints of the opposite sides. Where the medians are concurrent is called the centroid, this is labeled K. Therefore, since each median splits a side into two equal parts, we know
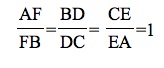
Each of the ratios is equal to 1 and so is their product. Therefore, the medians of a triangle are concurrent.
QED
Investigation 2:
Prepare a GSP sketch with script tool for a Rhombus in each of the 3 following situations:
(1) Given one side and one angle.
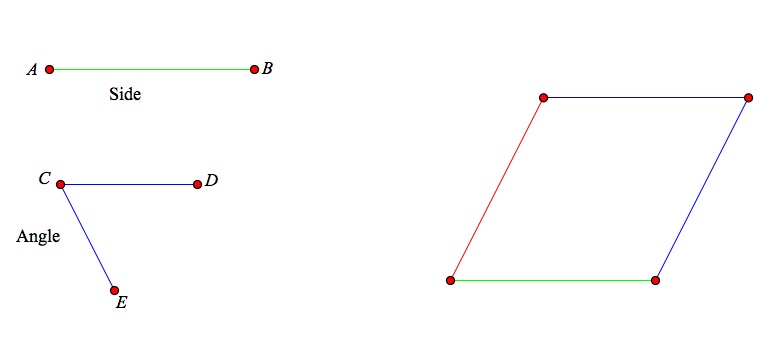
Click here for a GSP script tool.
(2) Given one angle and a diagonal.
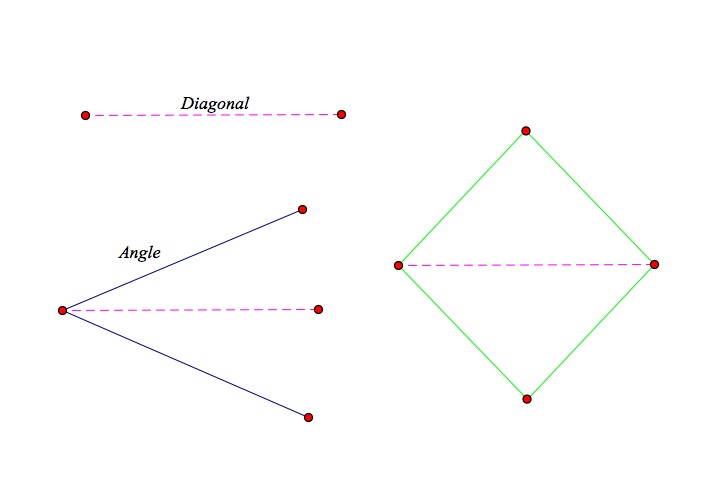
Click here for a GSP script tool.
(3) Given the altitude and one diagonal.
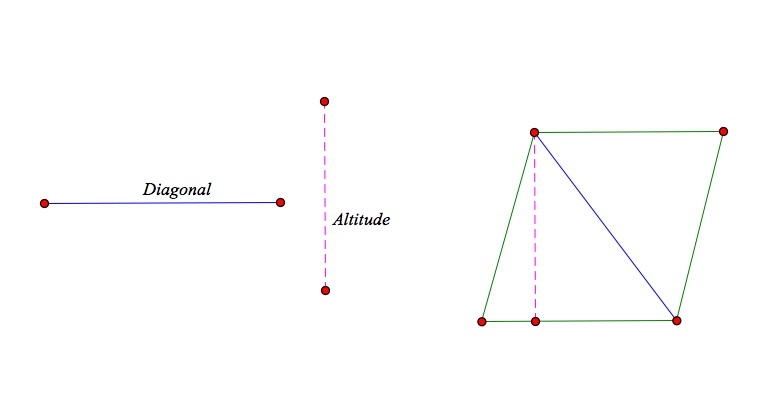
Click here for a GSP script tool.
For my additional write-up, I have chosen to continue explorations with the centers of a triangle from Assignment 4. Below, I will demonstrate and prove that the perpendiculars from a vertex to the line of the opposite side are concurrent.
As we know from Assignment 4, the perpendicular distance from a vertex to the opposite side of a triangle is known as the altitude of the triangle. Thus, we will prove that the altitudes of a triangle are concurrent, in other words, they meet at a single point, known as the orthocenter.
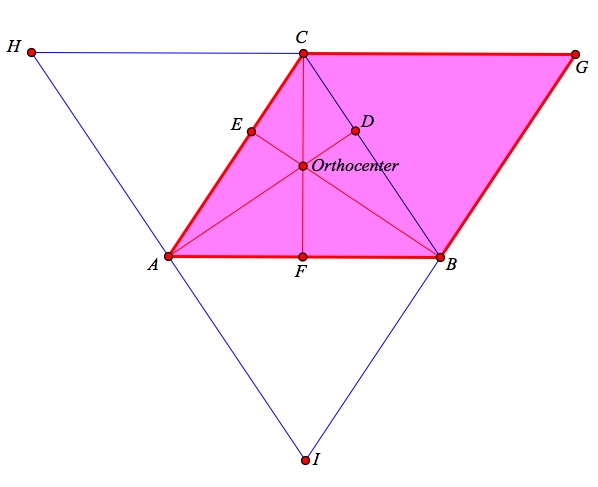
Suppose we have a triangle ABC with altitudes AD, BE, and CF drawn from the vertices of A, B, and C to the opposite sides BC, AC, and AB respectively. The points D, E, and F are the intersection points of the altitudes and the opposite sides of the triangles. Where the medians are concurrent is called the orthocenter. This is shown below:
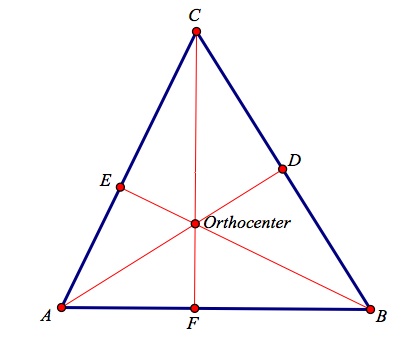
We must prove that the altitudes AD, BE, and CF are concurrent at a point P, known as the orthocenter.
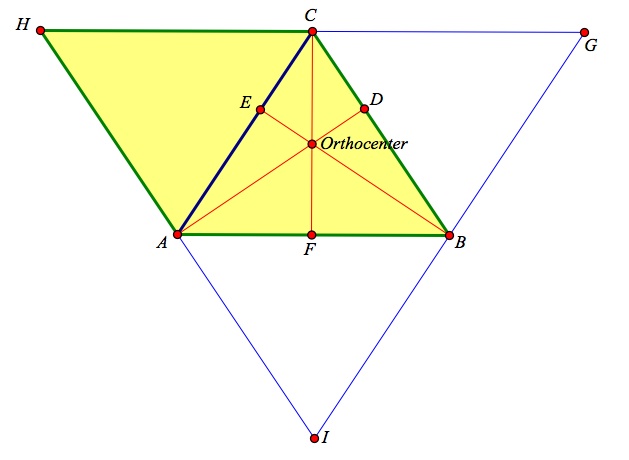
Begin by constructing a line that passes through the vertex C and is parallel to side AB.
By the similar way, construct a line that passes through the vertex B and is parallel to the side AC. Moreover, construct a line that passes through the vertex A and is parallel to the side BC. Label the intersection points of the constructed lines G, H, and I. This is shown below:
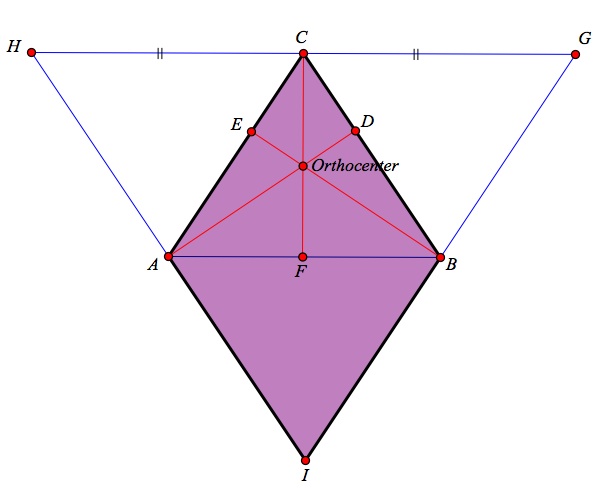
Now, we have a quadrilateral ABCH that has opposite sides which are parallel. Thus, its opposite sides are of equal length due to the properties of the sides of parallelogram(Opposite sides of a parallelogram are congruent and parallel - Notice where the parallelogram got it's name?!)
Thus, side AB and side HC are equal in length, so AB = HC.
Similarly, side AB and side CG are of equal length, so AB = CG (from the quadrilateral ABGC).
Hence, HC = CG, and the point C is the midpoint of the segment HG.
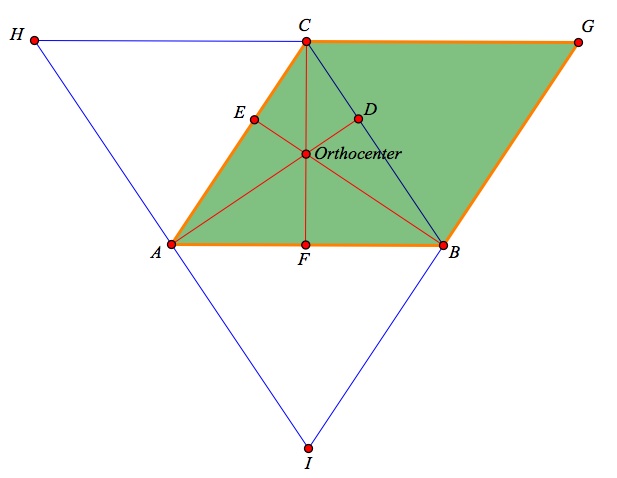
By the same reasoning, the point A is the midpoint of the segment HI. Thus, we can conclude BC = AH from the quadrilateral BCHA, and BC = AI from the quadrilateral BCAI, which gives AH = AI.
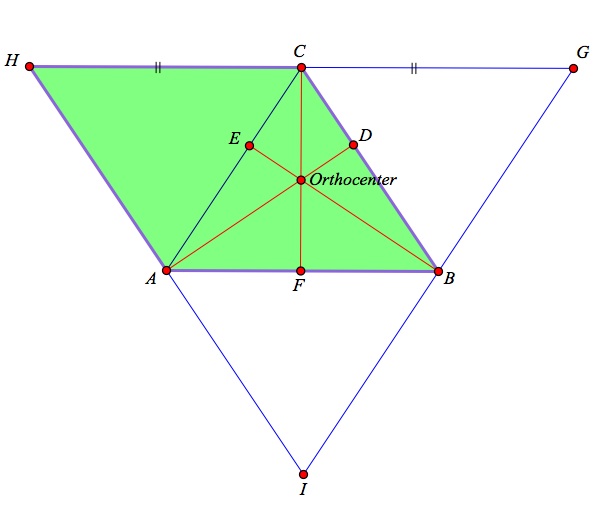
Finally, the point B is the midpoint of the segment GI. Thus, we can conclude AC = BG from the quadrilateral BGCA, and AC = BI from the quadrilateral IBCA, which gives BG = BI.
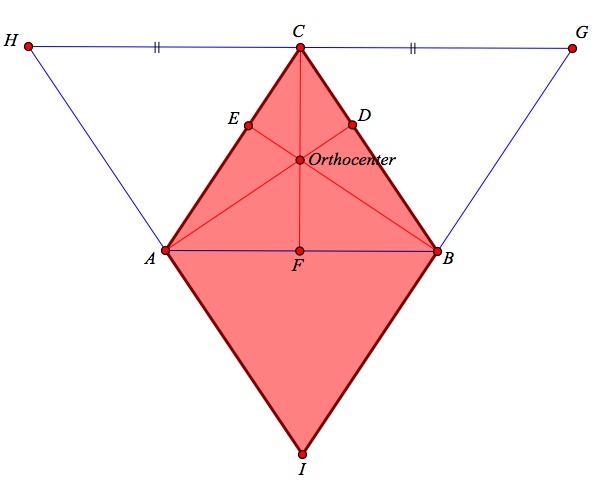
Therefore, the points A, B and C are the midpoints of the sides of the triangle IGH, and the altitudes AD, BE and CF of the original triangle ABC are the perpendiculars to the sides of the constructed triangle IGH at the midpoints of its sides.
Thus, the segments AD, BE and CF are concurrent at one point, P.
Click here for a GSP file of this construction.
This can also be proved by using the converse of Ceva's Theorem.Try it yourself!
QED